Category: Angle Theorems

Curious Colonies

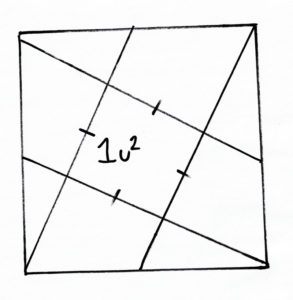
This science challenge requires an understanding of bacterial growth.

Diagram of the Curious Colonies

You receive an internship at Joana the scientist’s bacteria laboratory. Remember Joana from Pure Water and Spring Forward? She’s studying some exquisite bacteria. No bacteria colonies can grow over others, but they just block each other’s progress. Joana is studying them on a circular dish with diameter 1 metre. Zigzag bacteria colonies’ radii increase by 4 centimetre a minute. Linear bacteria grow at 2 centimetres a minute. Checkerboard bacteria grow at 5 centimetres a minute. Dotty bacteria grow by 1 centimetre every minute, and Inscribed bacteria grow by 3 centimetres a minute. Joana wants to display the results by simultaneously starting one colony of each type on the border of the dish. After some time, all the bacteria colonies will reach an equilibrium. The challenge is to determine where to place the colonies so that they will all end with an equal portion of the dish.

Solution

Salamandriform Sea

Geometry, calculus, and angle theorems are used in this challenge.

You are going on a vacation to an island in a sea. It’s a long journey. First, you have to drive to the sea. You park your car, take off your motorboat which you carried on top of it, and embark onto the sea. Then, you take your boat up to the island, park it, and walk up to your cottage. The sea and island are both ellipses, Whose foci are co-linear and point to your starting point. The sea and island both have their major axes twice the length of their minor axes, and you are a minor sea axis away from the closest point to the sea. Driving is 3 times faster than boating, which is thrice as fast as walking. The sea has only one island, and is 8 ninths water. What is the quickest route to your cottage?

Sophisticated Shapes

In this challenge, you will need to use geometry, calculus, algebra, and angle theorems.

A roulette is a shape created by rolling things. If you roll circles, you have a trochoid. If you draw the path of a point on the circumference, you have a cycloid. And if you roll that circle inside another circle, you have a hypocycloid. Simple enough. Say you have a circle with radius 25 centimetres and a circle with radius 1 metre. When you rotate the smaller circle inside the bigger one, the path traveled by a point on the circumference is a hypocycloid. It will touch the bigger circle 4 times every rotation. At these points, the instantaneous velocity of the point is zero. The challenge is to find the instantaneous acceleration if the small circle completes 1 revolution inside the bigger one each second. This is different from rolling completely over the inside, which it will do every 4.

solution

Crazy Capture

All of your algebra, geometry, trigonometry, angle theorems, and pathmaking skills are required to have a chance of solving today’s challenge.

2D capture example.

In a mathematical capture, all police officers and the target are points that travel at the same constant speed in infinite space in n dimensions. They have infinite endurance and perfect reaction time. The target is instantly captured and cannot ecape when a police officer touches it. In 1 dimension, when n = 1, it obviously takes 2 officers surrounding the target. When n = 2, we can do it with three easily. We do this by dividing the plane into three, each officer can easily trap the target in a 120° slice. The challenge is to see how many officers it takes to capture the target when n equals 3, in three dimensions.

Solution

Crazy Quadrilateral

You will need to use geometry, angle theorems, trigonometry, and your brain to solve this challenge.

Crazy Quadrilateral

We begin with a quadrilateral AEGH. We then draw a line from A to G to make ΔAEG and ΔAGH. ΔAEG is an isoscelese triangle. Next, we draw a line from point E to a new point, F, such that ∠AEF measures 60°. This makes a new point, D, where AG intersects FE. After that, we draw a line HC so that it is parallel to FE and intersects AG at point C. This makes ΔABC ~ ΔADE ~ ΔHAG. The challenge is to find ∠FEG if ∠HAG measures 90°.

Solution

Surprising Non-Congruency

Non-concruent triangles for the challnege.

Solving this challenge requires an understanding of geometry, trigonometry, and angle theorems.

In today’s challenge, we face two triangles who are not congruent. This is surprising because they appear identical, and we are told they share two sides. We can find that they also share and angle. However, because the angle isn’t between the sides, it’s only SSA. SSA is not a valid congruency theorem, because in some cases it gives two possible solutions. In this case, we have both of them, directly connected. The question is this: what is the length of the line that is cut unevenly? That is, the one whose length equals the sum of the sides that the triangles do not share.

Solution

Parallel Pentagon

In today’s challenge, you will need to use trigonometry, angle theorems, and geometry.

Diagram for today’s challenge.

The challenge for today is to find the area of the entire figure. We are given 3 lengths, 2 angles, 1 length equality, an angle equality, and finally, 1 set of parallel lines. Remember the units!

Solution