Author: zachary

Solution to Unbalanced Balance

Diagram of the now balanced balancing scale.

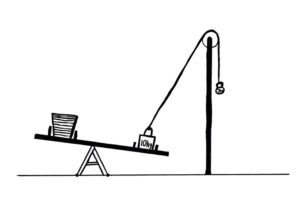
We’re trying to determine how much water must be poured into the bucket on the left to balance the balance. On the right is 2.5 metres of plank and a 10 kilogram weight. The weight is partially held up by a rope from a pulley. The weight opposite the pulley has a weight of 7 kilograms, exerting 7 kg * ≈9.807 m/s2 ≈ 68.65 mkg/s2 of tension on the rope. This lifts the 10kg weight, and so the force it exerts is equivalent to that of a 10-7 = 3 kilogram weight. It exerts a downward force of 3 kg * ≈9.807 m/s2 ≈ 29.42 mkg/s2. It is 2 metres away from the pivot, so it exerts ≈29.42 * 2 ≈ 58.84 m2kg/s2 of clockwise torque on the plank. Now, the plank on the right has a weight of 2.5 kilograms, so it exerts a downward force of 2.5 kg * ≈9.807 m/s2 ≈ 24.52 mkg/s2. It’s centre of mass is 1.25 metres away from the pivot, so it exerts a clockwise torque of ≈24.52 * 1.25 ≈ 30.65 m2kg/s2. The total clockwise torque is ≈58.84 + ≈30.65 ≈ 89.49 m2kg/s2. On the left, there is 1.5 metres of plank, weighing 1.5 kilograms. It exerts a downward force of 1.5 kg * ≈9.807 m/s2 ≈ 14.71 mkg/s2. It’s centre of mass is 0.75 metres to the left of the pivot, so it exerts a counterclockwise torque of ≈14.71 * 0.75 ≈ 11.03 m2kg/s2. Finally, water at 20° celsius has a density of 998.2 kg/m3. The bucket is 2 metres to the left of the pivot. The counterclockwise torque the water must create is ≈89.49 – ≈11.03 ≈ 78.46 m2kg/s2. This requires a downward force of ≈78.46 / 2 ≈ 39.23 mkg/s2. The mass required to exert this force is ≈39.23 / ≈9.807 ≈ 4 kg. The volume of water that has this mass is 4 / 998.2 = 4.007 × 10-3 m3. We have our answer. There is an easier way to do this problem, where we do not calculate gravity. This works because all the pieces that we start and end with do not use gravity. It makes sense because a scale would still work on the moon.

Loopy Lines

You will need to use geometry and algebra to solve this challenge.

Graph of Loopy Lines for this challenge.

We begin this challenge with 2 points. These points are at (1, 1) and (-1, 2). You may know that it only takes 2 points to define a line. You may have guessed that that is what we will use these points for. A second line is drawn, and it passes through the point (3, -2). A third line is then drawn, and it passes through points (2, 3) and (4, 0). A circle is drawn from the 3 intersection points. The radius of the circle is 5 units. The challenge is to find it’s centre.

solution

Unbalanced Balance

Physics is needed for this challenge.

Depiction of scene for Unbalanced Balance.

There is a lever. On one side is a bucket with negligible weight. On the other is a 10 kilogram weight. The bucket is a metre to the left of the pivot, the weight, 2 to the right. Lever has an additional 50 centimetres of unoccupied space. The weight of the lever is 1 kilogram per metre. The weight is attached to a string, and the string goes around a pulley. On the other side is a 7 kilogram weight. The challenge is to figure out how much water at 20° celsius must be poured into the bucket to balance the scale.

solution

Solution to Scintillating Structure

The volume of an ‘ice cream cone’ is that of the hemisphere, 2/3 πr3, plus that of the cone, πr2h/3. If the angle at the bottom of the cone is 30°, then we can make a right triangle with angle 15°. It’s height is r/tan(15°), or 3.372r. This makes the volume of the ice cream cone 5.372rπr3/3. This is 500ml, or 500cm3. We can then make the equation 5.372rπr2/3=500cm3, which solves to r=9.428. Now, the surface area of the hemisphere is 3πr2, and that of the cone, πr(r+√(h2+r2)). But we must subtract 2πr2 for the 2 circles that are not exposed. That leaves us with 2πr2 + πr√(h2+r2). Plug in r=9.428 and h=3.372r to get our answer. 1601cm2.

V=500cm^3=1.791\pi r^2\\[8pt] r=9.428cm\\[16pt] SA=2\pi r^2+\pi r\sqrt{h^2+r^2}\\[8pt] SA=2\pi r^2+\pi r\sqrt{1.07177r^2}\\[8pt] SA=5.732\pi r^2\\[8pt] SA=1601cm^2

Solution to Fascinating Frequencies 6

We’re trying to figure out the minimum amount of fuel that needs to be burned in order to bring a rocket back into geostationary orbit. Firstly, how much energy is released by the explosion? Sodium is an Alkali with atomic weight 23, and Fluorine is a Halogen with atomic weight 19. This means they are in the perfect proportions to explode. There is no precise amount of energy this explosion will produce. However, we’re trying to find how much fuel is necessary to ensure the scientists can get back to orbit. So we take the maximum. The first ionization energy of sodium is 4.26 eV. The electronegativity of fluorine is 3.98 eV. 4.26-3.98=0.28 electron volts of energy released per reaction. There are 6.022×1025 of each. The total energy released is 2.702×106 m2kg/s2 of energy. This produces a force of 2.702×103 metres kilograms per second. Divide this by the mass of the station, 500Mg, and you get a total acceleration of 5.404 ×10-3 metres per second of delta v. The most fuel efficient way to undo this is to simply wait until the rocket comes back and then switch back. This is because the rocket’s new orbit will still include the spot it changed orbit’s at, and in the case of prograde burns, this will be it’s perigee. The perigee also happens to be the time when retrograde burns are most efficient. The release of 5.404 ×10-3 metres per second of delta v requires a push of, guess, 2.702×103 metres kilograms per second of force, same as last time. This requires, again, 2.702×106 m2kg/s2 of energy. The liquid fuel used by the scientists, oxygen and hydrogen, releases 1.418 m2/s2. This does not seem to be the correct unit, it is missing the mass. That is because the mass is the unknown. We divide the total energy by this to find the total mass, 1.906×106 kilograms. But this weighs far more than the station! The scientists do not have enough fuel to get back into orbit.

Solution to Sophisticated Streetcar

We’re trying to figure out when you should jump off of a streetcar to arrive as soon as possible. The distance you need to run is equal to the square root of the sum of the square of the distance of your destination to the streetcar track and that of the streetcar track before the shortest run. The former is simply 1 million square metres. Let the second be x squared. The distance is then defined by √(1,000,000+x2). The point to jump off is thus when the derivative of this is equal to 5/15, or 1/3. This relationship is shown by the equations below and is true when x is 250√2. The equation gives 750√2 at that point, so divide that by 5 m/s to see how long you run for. Our answer is 150√2, or 212.1, seconds of running.

\frac d{dx}\sqrt{x^2+1\,000\,000}=\frac13\\[8pt] \frac d{dx}\sqrt{x^2+1\,000\,000}=\frac x{\sqrt{x^2+1\,000\,000}}\\[8pt] \frac x{\sqrt{x^2+1\,000\,000}}=\frac13\\[8pt] x=250\sqrt2

Fascinating Frequencies 6

For our final Fascinating Frequencies challenge, we will be using chemistry, physics, simulations, and orbits.

An experiment is held in space station orbiting earth from a geostationary orbit. Unfortunately, the scientists onboard forgot to properly secure one side of the space station. The side pointing prograde. Well, at least not retrograde, but their orbit is still messed up seriously. The reaction was between sodium and fluorine, with 19 kilograms fluorine and 23 kilograms sodium. The mass of the space station is 500 megagrams, including remaining fuel. How many grams of fuel must the scientists burn minimum to bring their rocket back to geostationary orbit? The scientists’ rocket fuses hydrogen and oxygen.

solution

Sophisticated Streetcar

Algebra, trigonometry, and calculus are used in today’s challenge.

You are taking a streetcar through a city. Your destination is in the middle of a large park, and you are late. You can jump of the streetcar at any time while it is on the side of the park. Your destination is 1 kilometre from the road. The streetcar moves at 15 m/s, and you move at 5 when you run, which you will to try to be on time. You calculate when you will need to jump off the streetcar to arrive as soon as possible, and jump of exactly then. For how long do you run?

Solution

Solution to Fascinating Frequencies 5

We’re trying to find the frequency at which a gear on a fidget toy is spinning. Firstly, the centres of each gear form a hexagon shape. Since each gear has radius 10 centimetres, this hexagon has side length 20 cm. In a hexagon, the distance between two opposite vertices is double that of the side length. So the outermost gear is 40 cm from the innermost. Centripetal force is π2rλ/s inwards. r is the distance between the point that’s moving and the point it’s moving around. λ is the frequency at which it is moving around. We can move around the elements around to see that λ = Fs/π2r, where F is centripetal force. Plug in the knowns to see that the gear is moving around 0.2533 times per second. If you tried making the device, you’d see that only three gears spin. That’s because, for the anchored gear to stay in position, the motion of the hexagon has to exactly balance it’s rotation. That means 2 other gears will be balanced, but the other 3 will move at double speed. Half from the rotation of the hexagon and half from their own rotation. So the rotation from the hexagon, 0.2533 rotations equals that at which the gear is spinning. Therefore the gear is moving at 0.2533 rotations per second.