Day: November 10, 2019

Solution to Captivating Circles BONUS

Free body diagram of cylinders illustrating why they do not roll.

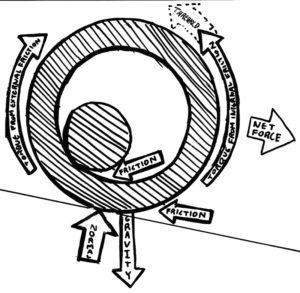
We’re trying to find the rate at which a cylinder rolls. This is actually rather simpler than you would think. For every metre the small cylinder rolls, the big one rolls three. The small one has internal friction with the big one. But, this is more than that of the big one and ground! The push of this friction is not only exerted by the big cylinder onto the small, but vice versa! Now, the cylinder would roll because of the counterclockwise torque applied by the friction with the ground. But the internal friction is so much stronger that it overpowers that torque with an even stronger clockwise torque threshold. Remember, it only happens when the counterclockwise torque is applied, so it is only a threshold. It will not cause the cylinder to roll backwards or anything crazy like that. This principle also applies to the normal force exerted by the large cylinder unto the small. Because of this, it is as if the small cylinder did not exist! Well, not really. But in a purely mathematical concept, it will be once equilibrium is reached. Knowing this, we need only look at the big cylinder. The acceleration gravity applies downward, combined with the normal force from the ground, exerts a total force dictated by the equation below. For 9.807 m/s2 and 15°, it gives 9.473 m/s2. Per metre, the kinetic friction is 1 kg/s. To equal the acceleration downwards, we divide, and get 9.473 metres per second.

\vec F_g-\vec F_n=F_g\cos\theta\\[4pt] =9.807\frac m{s^2}\times0.9659\\[4pt] =9.473\frac m{s^2}

Solution to Loopy Lines

Graph of Loopy lines for this challenge.

We’re trying to find the centre of a circle given the radius, a point on its circumference, and a point on a line that intersects 2 lines on its circumference. We already know one of the intersections. Since it takes 2 points to define a line, we can define 2 of the lines and find their intersection at (4.5, -0.75). Now, to find the centre of a circle, we draw the perpendiculars of 2 of the lines between the 3 points we are defining it with. Their intersection is the centre. We can use the 2 lines we know! That way, we know the slopes. Finding the intersection of 2 lines in y=mx+b form is easy peasy! x=(b1-b2)/(m2-m1), and y = m1x+b1. No problem. For the perpendiculars, we need the midpoints of the lines between intersections. No problem either, x = (x1+x2)/2, and y = (y1+y2)/2. To get a line given it’s slope and a point it passes through, we use b=y-mx. The slope is -1/m, where m was the old slope. The distance between 2 points is √((x1-x2)2+(y1+y2)2). Put all these simple things together and the answer should be easy. Let the slope of the line be x. Plugging x in through these equations gives the big equation below. A little bigger than expected. It equals 5 when x≈-2.954 and x≈-0.2364. These give the centres (5.503, 4.419), and (9.185, 0.9967).

\sqrt{\bigg(-{33x+166\over8(2x+3)}-2.053125\bigg)^2+\bigg(-{51x+214\over4(2x+3)}-{2x+3\over2x-3}-5.98125\bigg)^2}