You will need to understand calculus and linear algebra to solve this problem.
f(x) = g(x+1)-x,\space g(x) = f(2x)-2Today’s challenge is this: what is the derivative of f( g(x) )? Good luck!
Month: February 2019
Solution to Altitude Triangle
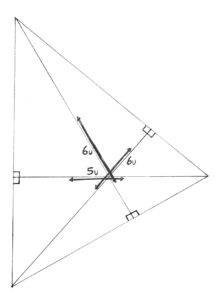
In this challenge, we’re trying to find the area of this triangle, with perimeter 20. Now, it is important to recognize that this is an isosceles triangle. That means that 2 sides are equal, and the third side is bisected. That means that if we let x and y be the side lengths, x+2y=20. Also, using the pythagorean theorem, x2 /2+25 = y2. The only solution to this is x=7.5, and y=6.25. That makes the area 5x/2, or 18.75 u2
Altitude Triangle
Todays challenge requires you to understand the pythagorean theorem, in the topic of geometry. You will also need to comprehend angle theorems.
The altitudes in a triangle measure 5, 6, and 6 units. The perimeter of said triangle is 20 units. The challenge for today is: What’s the area?
Solution to Perfect Square
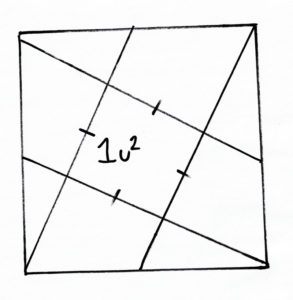
We wanted to find the area of a square! Now, the first thing we should take note of is that the small triangle is similar to the larger triangles because all of it’s angles are the same. If we let x and y be the 2 segments of the top line, x on left and y, right, then we can make an equation. specifically, find the area of the small triangle via hypotenuse comparison. The hypotenuse of the small triangle is y, and the hypotenuse of the big one is √(y2+(x+y)2). This makes y/√(y2+(x+y)2) equal one third, or (x+y)2=8y. To get this we simply raise both sides to the power of -2, subtract 1 from both sides, and then multiply by y. We also know that the area of the whole square is (x+y)^2, and that, since each outer region is a third of y(x+y), the small square is (x+y)2 – 8/3*y(x+y). That gives us the equation (x-5/3*y)*(x+y)=1. We can combine our 2 equations to solve for x and y. The positive, real number solution is x ≈ 1.120, y ≈ 0.2269, so the side of the square is ≈1.347, making it’s area 1.815 square units. And there’s our answer.